Satoshi Nakamoto, who created Bitcoin, probably owns 1.1 million Bitcoins.
If we calculate based on the recent price of Bitcoin at $60,000 per coin, Satoshi Nakamoto has $66 billion. So, the current richest man in the world is undoubtedly Musk. . .
Yes, Musk's net worth is about $210 billion. Even if you have 1.1 million Bitcoins, it's still less than one-third of Musk's.
But the problem is, Musk's money, he won't give you a penny...
You may be amused: "How can Satoshi Nakamoto's money be given to me?"
Hey, if he doesn't give it to me, you can take it yourself!
Strictly speaking, if you take Musk's money, you will definitely break the law; but if you take Satoshi's coins, you will not break the law.
I have some time recently, so I will make a tutorial to teach you how to take Satoshi's coins. Let's get started!

(I) The "256 Doors" that Let You Sleep Peacefully
If you want to take it, you must first know where the coins are.
Bitcoin is an "encrypted digital currency". I know that many people are a little confused when they hear "encryption" and think that only academic masters can understand it.
It should be said that if your goal is just to "take away Satoshi's Bitcoin" instead of creating a complete Bitcoin system like Satoshi, then I can tell you responsibly: You don't need any encryption knowledge at all.
Because in the world of Bitcoin, all the money is just there, as long as you *go and take it*, it's yours. Is it a bit confusing? Is this talking about Bitcoin? Why is it different from what others say? In fact, it's the same, but many people haven't *explained* the principle of Bitcoin to you from the beginning.
Everyone likes pie in the sky, let's start with a cool scene:
After a hard day's work, you picked up a lunch box on the way home from get off work, opened it, and it was full of gold coins.

What would you do?
I guess many people would make the same choice: keep it to yourself and hide the coins somewhere.
Then comes the real challenge: where to hide?
Under the bed? In a large wardrobe? In a safe? In a microwave? In a cake box? In a hole in the yard? Put a dead dog on top and plant a rare plant on top?
Of course, all of these places are OK, but there is a certain probability that others will find it, but the probability varies. If you put it in any of these places, you can't be 100% assured, and you will definitely not sleep well at night.
In fact, when Satoshi Nakamoto envisioned the Bitcoin building, he first had to solve this "foundation" problem:
Can we design a space where you, me, and him can safely hide gold coins?
If we use heavy reinforced concrete to build such a world, the cost must be huge. So, Satoshi Nakamoto chose something that has no weight at all - numbers.
What does this digital world look like?
Let's continue to imagine:
You are holding a small lunch box in your hand, and there are two doors in front of you, with 0 written on the left and 1 written on the right.
You have to choose one of the doors to go through. No pressure, no rewards or penalties, just choose randomly. Let's say you choose 1.

After going through door 1, you see two more doors, with 0 on the left and 1 on the right. You have to continue to choose a door to go through.
After going through this door, there are two more doors.
And so on.
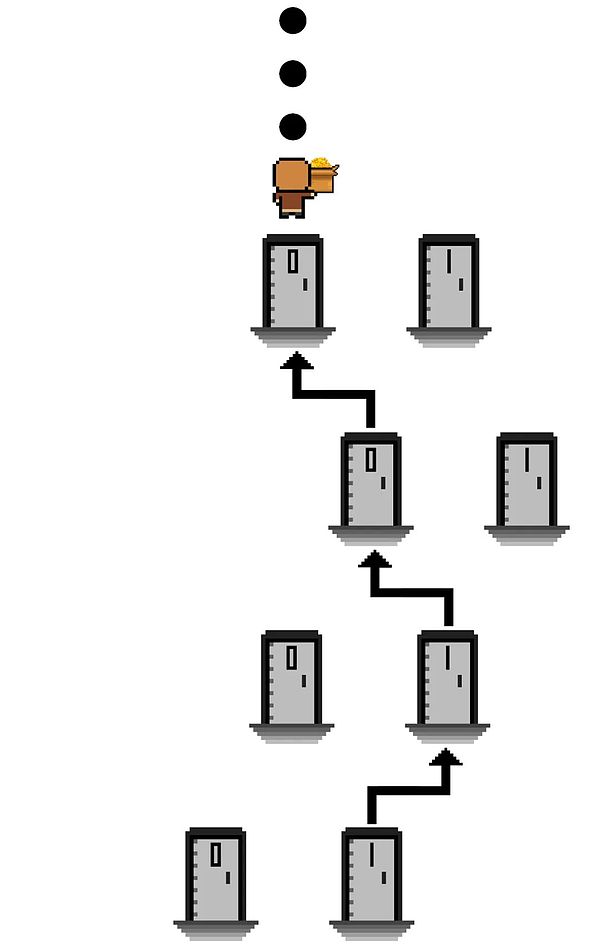
When you pass through the 256th door, a planet appears in front of you.
The planet is not big, just like the one in "The Little Prince", where you can watch 44 sunsets a day.

You can hide a lunch box of gold coins on this planet.
Actually, it's not hidden, just put it on the ground.

Then you turn around and return to our world, point at your boss's nose and scold him, and finally write a resignation letter saying "The world is so big, I'm going to see it" and throw it in his face.
When you want to use these gold coins to enjoy life, you only need to push open those doors again, go to your planet to get a gold coin or half a coin and sell it, and that's it.
With these "256 doors", you can achieve sleep freedom, sleep whenever you want, and sleep to death.
As long as others don't pry open your head, they won't know that you have gold coins, let alone where your gold coins are.
Of course, there is a key problem here: you have to remember the order in which you pushed open the 256 doors!
If you forget and push even one wrong door, you will not arrive at the previous planet, but another one that looks similar, but without your lunch box on it. . .
If you never remember, your gold coins will remain on the previous planet forever. Although others can't get them, you can't get them either.
So to be safe... it's better to write down the "order of the doors" on paper.
What you wrote on the paper is probably a string of numbers: 001101011101100101.... (256 bits in total)

Now the problem becomes: your note cannot be peeked by others. Otherwise, others can also take your gold coins.
Essentially, your door is not "locked" and you don't need a key.
If you have to say it, this order of opening the door is your key.

The above is actually the basic principle of Bitcoin storage - it does not use passwords, but uses simple mathematics to imagine such "256 doors" and "planet as numerous as the sands of the Ganges".
Do you realize something?
Aren't the Bitcoins of Brother Satoshi Nakamoto placed directly on a planet?
In other words: as long as you open the door randomly and try different orders, you may happen to encounter the same order as Satoshi Nakamoto! At this time, you can board his star and take the Bitcoin away.
Just get all 256 correct answers~~
How about it? Although it’s a bit difficult, it seems there is still some hope, right?
And according to research, Satoshi Nakamoto’s Bitcoin is not only placed on one planet, but scattered on hundreds or even thousands of planets. As long as you get any planet right, you can get something! Isn’t the success rate greatly increased?
What is the success rate?
Since everything is built on mathematics, the probability can be calculated. Let’s calculate it.
(II) Be a “gambler” in the Bitcoin universe?
Each time you have 2 options, you have to choose 256 times in a row, so there are a total of 2²⁵⁶ possible situations.
Do the math, this number is:
115,792,089,237,316,195,423,570,985,008,687,907,853,269,984,665,640,564,039,457,584,007,913,129,639,936
How big is this number? It is approximately equal to 1 followed by 77 zeros.
This is roughly the number of atoms in the universe.
You might as well understand it this way: Bitcoin uses mathematics to create a "magic" that turns every atom in the universe into a planet.
You log in to a planet at random, and it is very unlikely that it happens to be logged in by another person.
To put it bluntly, if you use the world's fastest computer to traverse and try one planet after another, if you want to land on Satoshi Nakamoto's planet with certainty (if you are unlucky), it will take at most billions of years.
Here is the concept of "information entropy".
The so-called information entropy can be understood as the difficulty of information being "blindly" guessed. The easier it is to guess, the smaller the information entropy.
And the "Bitcoin universe" designed by Satoshi Nakamoto has extremely large information entropy.
But even so, if you are lucky enough, you may still land on the planet Nakamoto by chance on your first try!
Even if the king of heaven comes, the probability of such a big luck is not! Zero! !

Speaking of this, do you have the urge to try your luck?
No problem, let's do it in practice and experience the excitement of gambling.
To be clear, our goal now is: select 256 numbers, and then enter them into the Bitcoin wallet program to check if there happens to be a balance.
Begin below.
Step 1: Sprinkle the flour.
Actually, you can just write 256 numbers composed of "0, 1". But for the sake of professionalism, let's do a ritual.
You find a dice? Throw it. If it is 1, 2, or 3, write 0. If it is 4, 5, or 6, write 1. How about it? It has a bit of "Feng Tian Cheng Yun" flavor, right?
For example, it can be written like this:
left;">01000011111101011101100100100000101001101000100001000111000101101100100110111110100000111100100000110111110010000101010 00001011 101100110000110111000010011011011110011100101011110011010000010011100000100100000111001110011110101110001
This string of numbers is like a spoonful of flour you scooped at random, with the flour particles in it arranged randomly in various postures.
Oh, and pay attention to the format when writing. Wrap the line every time you write 11 numbers. Writing 256 means you will write the third number on the 24th line.

Hey, why do you write it like this? Why are there 3 more numbers in the 24th row? How can people with obsessive-compulsive disorder live with this?
Actually, there are not 3 more numbers, but 8 fewer numbers.
The last 8 numbers require us to calculate the first 256 numbers to get them - its scientific name is called "checksum".
Step 2: Knead the dough.
We need to perform an operation called SHA-256 on the first 256 numbers.
You can think of SHA-256 as an equation. Any number entered into it will give a result, and this result is very regular, and it must be a binary number with a length of 256 bits.
This equation is very complicated, full of various mixtures and repetitions.
It is a bit like kneading dough. The flour that was originally separated becomes a whole dough after repeated kneading.
This dough is called "hash".

Of course, kneading dough in our real life is very casual, and the same flour kneads different dough every time.
But mathematical formulas are very rigorous. As long as the initial value remains unchanged, the result "kneaded" by the SHA-256 algorithm will always be the same.
One more thing, since it is an equation, you can calculate it by hand.
There is a stubborn brother who actually calculated SHA-256 by hand. I posted the link at the bottom. Students who are interested in the specific calculation steps can go and see the brother's self-torture process.

As a human being in the 21st century, I sincerely suggest that you use a computer to calculate...
If you have a Mac computer, you can open the "Terminal", then copy the following command into it, and you can get the result by pressing Enter.
echo 010000111111010111011001001000001010011010001000010001110001011011001001101111101000001111 00100000110111110010000101010000010111 011001100001101110000100110110111100111001010111100110100000100111000001001000001110011100111101011100011001110101101110001 | shasum -a 256 -0
If you are using a Windows computer, it is not very convenient. You can just let ChatGPT help you calculate it.

If you calculated it correctly, the hash value you got must be this:
3F38F4FC5FB00C2A6D0648B16E4D67C206D055524163BDADF4BBD288931AE51D
This is the hexadecimal form, and you still have to let ChatGPT convert it back to binary, which is the following:
00111111001110001111010011111100010111111011000000001100001010100110110100000110010010001011000101101110010011010110011 11100001 0000001101101000001010101010100100000101100011101111011010101101111101001011101111010010100010010010011000110101110010100011101
Step 3: Get out of the pot.
We pick out the first eight digits of this long hash sequence, which is 00111111.
Add it to the end of the previous random number to get a 264-bit complete sequence:
left;">01000011111101011101100100100000101001101000100001000111000101101100100110111110100000111100100000110111110010000101010 000010111011 0011000011011100001001101101111001110010101111001101000001001110000010010000011100111001111010111000100111111
OK, your private key is ready to be served!

It should be emphasized that although this number has 264 bits, for you, it has the same "information entropy" as the previous 256 bits.
Because once the first 256 numbers are selected, the next 8 numbers are determined, and there is no information increment.
But if you accidentally copy the previous numbers incorrectly, the checksum will change, and the Bitcoin wallet can remind you that you have entered the wrong number - this is the meaning of "checksum".
Seeing this, the most difficult part is actually over.
The next step is to verify whether we have bet on the "prize opening moment" of the Nakamoto wallet!

(III) Good news for the oblivious: mnemonics
Just now I asked you to write 11 numbers each.
Because every 11 numbers actually corresponds to a word.
It is said that after Satoshi Nakamoto disappeared, there are still some technical experts who spontaneously maintain the Bitcoin system. They continue to put forward "improvement proposals" to contribute to the Bitcoin system.
Among them, the most awesome proposals are worthy of being honored by the Imperial Ancestral Temple - they can get the BIP number (Bitcoin Improvement Proposals).
The BIP-39 we are going to talk about next is the 39th important proposal in the history of Bitcoin. Its content is:
It is too hard for everyone to remember so many 0s and 1s, and it is easy to copy them wrong. It is better to map every 11 binary digits into a word as a group.
There are a total of 2048 possible permutations of 11 binary digits, which means that as long as 2048 words are selected, all situations can be equivalently marked!
If you check the original text of the BIP-39 proposal, you can easily see this list. I put the link at the end. Here I will also take a full screenshot for you to see. Click on the chicken to enlarge it:

Next, you need to look up this table, find the English words corresponding to each row of numbers, and then write them down, a total of 24 words.
Specifically, the string of numbers just now is equivalent to these words:
dumb put else escape love merge cheap spare sight salad bench conduct giant second hundred slab old evoke drastic attack pact shoe punch child
This is your "mnemonic".
Note that the "information entropy" of this mnemonic is the same as the 256 words you initially selected by rolling the dice, and they can also be converted to each other.

Although the sentences made up of these words have no practical meaning, aren’t they much easier to remember?
In addition, the Bitcoin Core Team is very friendly to China, and has also provided two Chinese mnemonics schemes in Simplified and Traditional Chinese in BIP-39, each of which is 2048 characters. Here is a screenshot for you to see:
In essence, it doesn’t matter what language you use. As long as the numbers are the same, the functions of Chinese characters and English words are the same.
It’s just that in reality, everyone is still used to using English, so we should follow the local customs.
Next, let's download a digital wallet that supports Bitcoin and enter these 24 mnemonics.
The prize draw time is here.
Dangdang! The balance of Bitcoin on this planet is - 0.
Don't be sad, if you understand the previous probability, you will know that this result is not surprising.
The vast majority of planets in the Bitcoin universe are so desolate - in the turbulent cosmic wind, someone can come and stop for a few seconds, which is already a very romantic encounter.
If you cherish this fate and don't want to say goodbye to this planet, you can come back often to take a look.
Of course, if you want to let this fate develop a little bit, you can... Get some Bitcoins and put them on this planet...
I described the whole method as "gambling" before, because we want to use it to "encounter" Satoshi Nakamoto's planet. But the actual effect of this method is to let you encounter your own planet.
You can't find Satoshi Nakamoto's private key, and others certainly can't find your private key. Isn't this "planet" exclusive to you?

Just like the little prince, he lives on an asteroid called B-612.
There are two active volcanoes and one extinct volcano on the star, some caterpillars, wind, and some weeds. In short, it is an extremely ordinary star.
But the little prince's home is there, because there is a rose on this small planet.
With this rose, B-612 is instantly different from all other stars for the little prince.
This is the process of "meaning" emerging.

Of course, if Bitcoin only has this level of meaning, then it is just a fable like "The Little Prince".
The reason why Bitcoin is known, used and discussed by so many people is that it can be "moved from one planet to another".
That is, the ability to transfer.
In fact, in order to achieve the ability to transfer money, it must be attached with a whole set of sophisticated modules, and the so-called "encryption" also starts from this step.
If you successfully land on Satoshi Nakamoto's planet, then how do you transfer his coins to your own planet?

(IV) From Door to Window: Bugs in Card Universe
As long as it is called "transfer", it must have a basic characteristic: I can actively transfer it to you, but I cannot force you to transfer it to me.
It is an irreversible one-way ability.
One-way ability is generally based on "one-way structure".

Let's design this structure step by step:
If I want to put 1 Bitcoin on the Little Prince's planet, I certainly can't let him tell me how he chose these 256 doors, that is, the "private key".
Because once I know his private key, I can not only transfer the currency to him, but also transfer his currency to me.
This is reversible and does not meet the requirements.
Is there a way to locate the Little Prince's planet without revealing his private key?
The method used by Satoshi Nakamoto is very clever-the Little Prince does not need to show me his "door", but only his "window".
The "elliptic curve algorithm" is used here. (Specifically, it is one of the elliptic curve algorithms: secp256k1)
Imagine a curve in front of you, and this graph corresponds to an equation. The equation looks like this:
y²≡x³+ax+b(mod p)
Here p is a very large prime number.
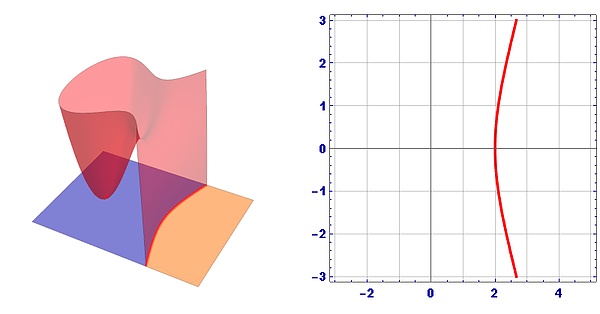
The formula is not important. You just need to understand the key point here. An elliptic curve with prime number characteristics has a special "personality":
The coordinates of point A can be easily deduced from the coordinates of point B after x transformations,
but the coordinates of point B and point A cannot be used to deduce the number x.
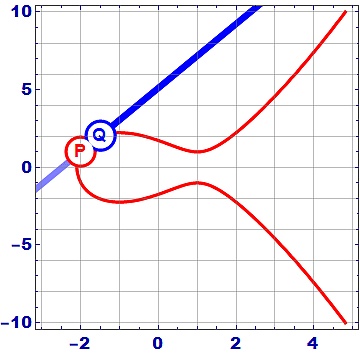
This picture shows an elliptic curve, and P in the picture is the coordinate of point A. You can connect points P and Q to get R, and then you can use point R as the new point Q and connect it to P, repeat x times, and finally get the coordinate of point B.
After all, it is the crown jewel of cryptography. Elliptic curves are a bit difficult to understand. I found a good reference material and attached it at the end.
Here you just need to know: this property just meets the requirement of "irreversibility".
Now we define a fixed point as point A, and then take the private key in hand as x, can't we deduce the coordinates of point B?
I just use the coordinates of point B as the logo of my planet, isn't it?
Hey, this coordinate of point B is also called the public key corresponding to our private key.
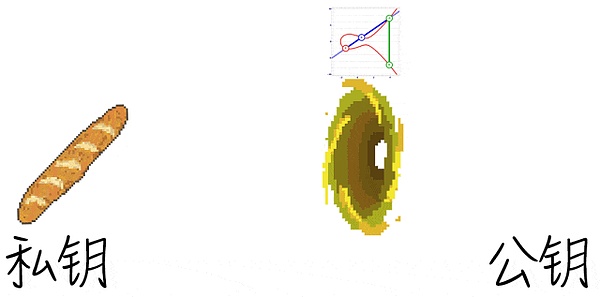
The private key becomes the public key, just like bread becomes a hot dog.
Although the names of "private key", "public key" and "elliptic curve" are high-sounding, in essence, this is that mathematicians first discovered a bug in the universe, and Satoshi Nakamoto invented a system to madly block this bug.
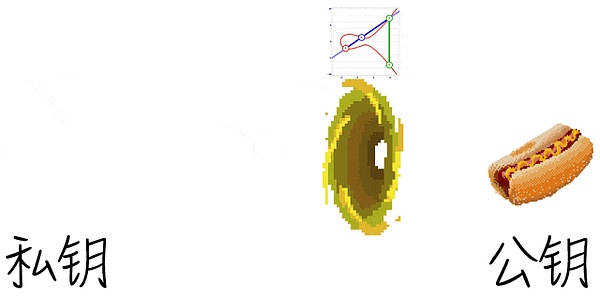
It is impossible for a hot dog (public key) to turn back into bread (private key).
Then I want to transfer money to the little prince, can he tell me his public key? Absolutely not! ! !
When Satoshi Nakamoto designed Bitcoin, he had a plan in mind:
The elliptic curve algorithm now looks like a cosmic bug. What if the bug is fixed one day in the future? What if a mathematician develops a reversible method? Wouldn’t that be the end?
So he finally decided to add two layers of irreversible hash operations (the first layer is SHA-256, the second layer is RIPEMD160) on the basis of the public key, and finally unify the format (using Base58check encoding) to calculate a string of numbers that even his mother wouldn’t recognize.
If hackers want to crack the Bitcoin system, they have to crack both the elliptic curve and SHA-256 cosmic bugs at the same time, which is extremely difficult.
(It turns out that this operation also brings unexpected benefits to the Bitcoin system, which we will talk about later.)
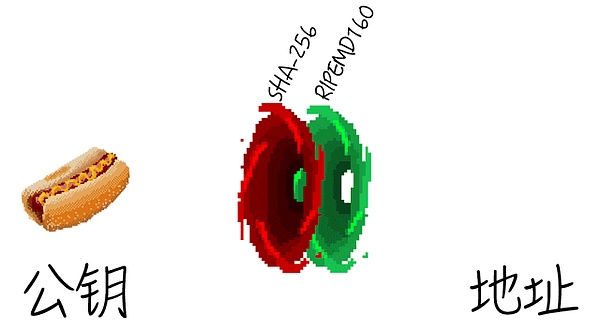
The public key is transformed into an address, just like a hot dog is transformed into a meal.
This number that even your mother doesn't recognize is called your "wallet address".
Let's summarize:
1. Each wallet address corresponds to a public key, and each public key corresponds to a private key. In other words, when you choose a private key, your public key and address are already determined.
2. However, others cannot infer your public key even if they get your address, and they cannot infer your private key even if they get your public key. Your coins can only be controlled by the person who holds the private key (yourself).
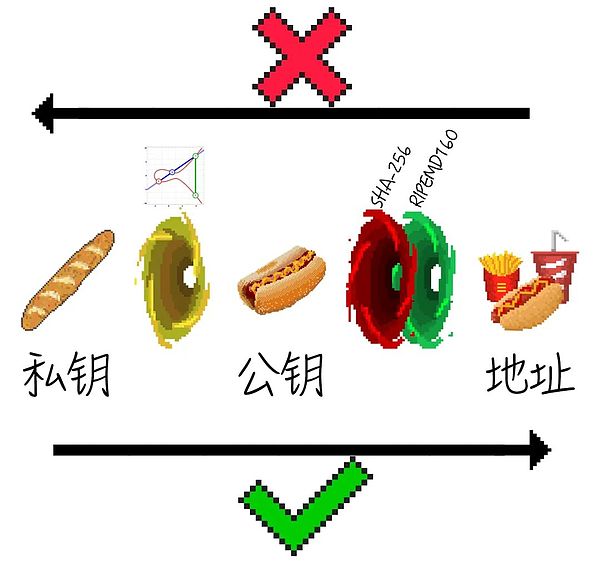
If we go back to the metaphor of "The Little Prince", it is:
1. Each planet has not only a door, but also a window. However, to get from the door to the window, you need to jump through a one-way wormhole composed of several mathematical puzzles. You can walk from the door to the window, but you can't walk from the window to the door.
2. The coin must pass through the door to leave the planet, but the coin can enter the planet through the window (it is even better to pass through the door).
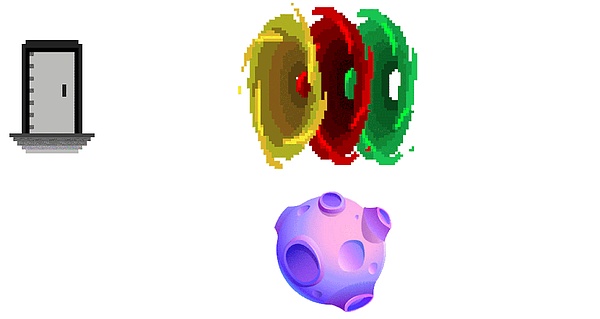
3. Since all the windows are open, everyone can "put coins" in through this window. Of course, they can only put coins in but not take them out, just like a merit box.
4. And the windows are transparent, and everyone can see inside. In other words, as long as you know an address, you can see how many coins are in it. Not only that, every time there is a coin in and out in history, the amount and time are all recorded.

How about it, is this design ingenious?
But do you feel that there is a new problem here?
I call it the “visual espionage problem”.
Although no one can move the coins on your own planet, everyone can see them!
It’s like you are sleeping in your own home. Although no bad guys come in to disturb you, the walls are made of glass. You can’t stand everyone visiting you while you are sleeping. . .

And it’s terrifying to think about it. These account movement information (amount, time) may also reveal the identity or time zone of some planet owners.
Imagine this:
You finally hit the cosmic level of dog shit, found Satoshi Nakamoto's wallet, transferred the coins to yourself, and became a Bitcoin tycoon.
But some bad guys use these account information to locate you in real life, and use the most efficient cracking method - guns - to force you to hand over your private key.
Then, no matter how exquisite the design of "doors and windows" in the mathematical world is, will it be of any use?
Satoshi Nakamoto thought of this problem a long time ago, and his solution is "disposable planets".
This is a genius idea, but in terms of the specific implementation plan, it is a little "off-standard".
Let's take a look.
(V) “Sub-throw Planet” and “Secret Thread”
Suppose: I have 2 coins on “Planet A” and now I want to transfer 1 coin to the Little Prince’s planet.
At this time, I must first find another planet, let’s call it “Planet B”.
The Little Prince will tell me his “planet address”. Then I initiate the transfer.
Note! At this time, both coins will go out of the door of my Planet A, one of them will fall into the Little Prince’s planet through the window, and the other will fall into “Planet B” through the window.
The coins have all run away from Planet A, which looks very wrong! But if you think about it carefully, you didn’t lose a penny of the coins left, they just landed on Planet B.
And you also know how to get to Planet B. After all, Planet B is the same as Planet A, and you chose it yourself!
The design of this "one-time planet" is called UTXO (Unspent Transaction Output), which means "unspent transaction output" in Chinese, which means that the money that is not spent must also be output.
In this way, all the money is "money that looks like it has never been spent". (A bit confusing, you can figure it out)
Its benefits are obvious: if a bad guy wants to track the flow of funds at a certain address, it will be difficult for him to determine which is the receiving planet and which is the change planet.
Of course, there are still deep rules in human behavior. If we use big data, we may find clues. We can find them in 《Bitcoin Pursuit》 and The Dark Web: Life and Death Tired tells the story of how this technology split the darkness and saved the world.
But after all, it is much more difficult to track. Unless you are a heinous villain, no one will spend so much money to analyze your Bitcoin capital flow.
UTXO is a genius design, but it has a very inelegant feature, that is, every time you transfer money, you have to change a planet (address) and re-record the private key. If you lose the last private key, your coins will stay on that planet forever. . . This is too creepy! !
Satoshi Nakamoto meant that if you want to be safe, you have to do it this way, don't talk nonsense.
In the Bitcoin wallet software he designed, there are 100 planets reserved, that is, 100 private keys can be placed, and one will be invalidated for each transfer. After 100 are used up, you have to put in 100 more.

It's complicated, right?
Fortunately, the real good things will eventually evolve!
After Satoshi Nakamoto disappeared, the subsequent developers of Bitcoin found a particularly elegant method, so that you only need to remember planet A to calculate the position of planets BCDEFG.... according to an algorithm.
This is the famous BIP-44 proposal.
The method in the proposal is actually very easy to understand:
First, you have a 256-bit random number, which is your private key to enter Planet A, right?
Then, you have to select another 256-bit random number, which is called the "chain code" of Planet A.
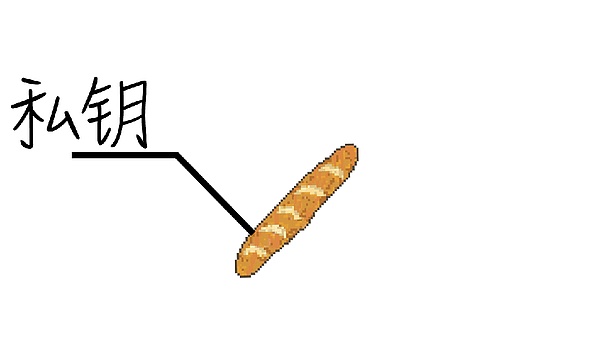
Finally, you need to use the "secret key derivation algorithm" studied by the Bitcoin core team. This algorithm requires three things to work: private key, chain code, and serial number.
You already have the first two, what is the serial number? You can simply understand it as: 1, 2, 3 in this order.
If you input these three into the derivation algorithm, you will get a private key of "Planet B" and also a chain code of "Planet B".
For a fixed planet, the private key and chain code are always unchanged. But you can change the sequence number at will.
So as long as you set the sequence number to 1, 2, 3, 4, 5, you can derive five planets B, C, D, E, and F for Planet A. They are the "child planets" of Planet A.
If you go to Planet B and use its private key and chain code to derive five planets G, H, I, J, and K, they are the "child planets" of Planet B and the "grandchild planets" of Planet A.
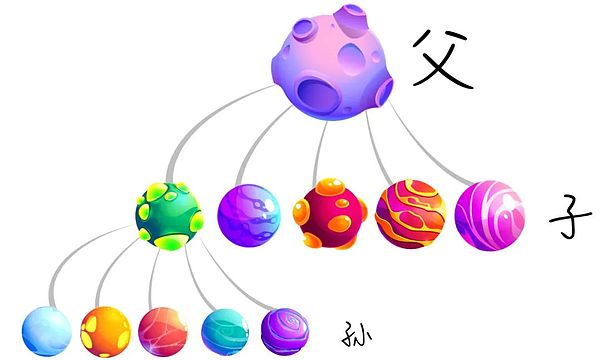
By analogy, you can derive countless descendants of planets with only Planet A and its chain code.
This trick is called "layered deterministic wallet" - HD Wallet.
You see, in this case, you only need to remember two strings of numbers (private key and chain code), which is much better than Satoshi Nakamoto's solution, right?
But then again, you still need to remember two strings of numbers. . .
The old irons in the Bitcoin developer community felt that it was not enough. They brainstormed and came up with a better solution!
(VI) Billions of “Private Planets” Belonging to You
They used a “secret key derivation” algorithm: using 24 mnemonics to derive the private key and chain code of Planet A.
In this case, the effect is explosive:
1. You still only need to remember 24 words;
2. But you can control the private keys of countless planets!
Note that in this case, these 24 mnemonics are not equivalent to your private key - mnemonics can derive private keys and chain codes, but private keys and chain codes cannot be deduced from mnemonics.
Think about it, this system has a magical feature:
To outsiders, all planets are the same, but from your perspective of the "dark side of the moon", it is like Spider-Man, with countless hidden threads connecting these planets.

This hierarchical determination model has many very practical scenarios. Let me give you two examples:
1. Due to the one-way push-down feature, the parent planet is a pure "scumbag". It can determine which are its own child planets, but the child planets do not know who is their parent planet.
That is to say, the parent planet can move the coins on the child planet at any time, but the child planet cannot move the coins of the parent planet.
In this case, as a Bitcoin tycoon, you can hire some younger brothers, each of whom helps you manage some assets.
You only need to give them a private key of a child planet. For the convenience of management, they can also use this child planet to derive grandchildren, etc., but if you are not satisfied with their work one day, you can directly take back the coins in their hands. (Of course, in this case, it is best to use a multi-signature wallet, which is equivalent to dividing the private key into several parts. I will not go into details due to limited space.) 2. The child planets do not know each other's existence. Since the calculation of the child planet requires the "parent private key", "parent chain code" and "serial number", the child planet does not know these three elements. From the perspective of the child planet, it exists alone and has nothing to do with anyone.
The ingenuity of this design lies in "minimizing the relationship chain":
1) Even if the bad guys know the public key of one of your planets, they cannot figure out which planets it is in a brotherly relationship with;
2) Even if the bad guys steal the private key of one of your planets, because they don't know the chain code, they can only move the coins on this planet, and cannot move the coins of its subordinate planets;
3) Even if the private key and chain code of a planet are leaked, the bad guys can only move the coins of its subordinate planets, and cannot move the coins of higher-level planets. However, there is a small problem here. If the public key and chain code are used, it is possible to deduce the public keys of all sub-planets. Although only the public key cannot move the account, it can understand the genealogical relationship of all sub-planets.
Do you remember the suspense we kept before?
Due to Satoshi Nakamoto’s cautious design, he did not use the public key directly as the address, but added two layers of encryption to generate the address.
This adds another black hole in front of the “public key”. Even the public key cannot be deduced from the address, which makes it impossible for hackers to reverse the “relationship between layered wallets”.
Speaking of this, I guess you also understand that the reason why the Bitcoin mechanism is extremely safe is that the most important places are all guarded by “crypto superheroes”. Some of the most critical places do not even have one hero, but a whole “Avengers”.
This security mechanism can protect your coins, and naturally it can also protect Satoshi's coins. Therefore, even if the so-called "method" is here, the probability of you cracking Satoshi's wallet is at the "universe destruction" level.
You must know that I wrote this article not to help you crack Satoshi's wallet, but to take you on a journey of "failed cracking" and to let you experience the full and stunning mathematical beauty in the Bitcoin universe.
Value comes from consensus, whether it is gold, legal currency or Bitcoin.
But in the end, I don't want to discuss the value of Bitcoin at all. What I want to discuss is the value of those "planets" used to store Bitcoin.
Maybe you don't necessarily resonate with me, but I really want to try.
(VI) Important things are invisible to the eyes
Let us return to the fairy tale "The Little Prince".
There are 8 planets in the story of the Little Prince: the planet where he lives, the six planets he briefly visited, and the Earth he finally visited.
What is so special about these planets?
In the eyes of others, they are not special at all, and there is no difference between them. But the Little Prince would not agree.
He arrived at these planets one after another, and met different creatures on these planets and interacted with them. These memories are personal, and the information they carry is real to the Little Prince himself.
It is like there is a "chain code" exclusive to a certain soul, which defines his life trajectory in the dark.
As the fox the little prince met said:
"To me, you are nothing more than a child, no different from thousands of other children. I don't need you. You don't need me. To you, I am nothing more than a fox, no different from thousands of other foxes. But if you tame me, then we will need each other. You are unique to me, and I am unique to you..."

There is a garden on the earth with 5,000 roses in full bloom. The flowers are gorgeous, but they are different from the little prince's roses.
If we trace back, a rose seed randomly floated to the little prince's planet B-126, and that was the beginning of everything.
What's important is that ordinary people like you and me are just like the little prince.
We are equal and indifferent, but we have our own foxes and roses. And this specialness may only come from a seed that no one can know in advance, perhaps - 256 dice rolls.
The eyes cannot see the truly important things.

The writer Chen Chuncheng wrote a short story, "Zhufeng Temple".
During a special historical period, the abbot of Zhufeng Temple hid the "Chendie Stele", the treasure of the temple, in the night to prevent it from being destroyed.
Since then, the stone tablet seems to have evaporated from the face of the earth. Even though history has changed its tune, it continues to remain silent and never reveals its true appearance.
Decades later, "I" came to stay in the temple. Because my old house had been demolished, there was only one key left, as a mysterious connection with my childhood time and space. I selfishly wanted to find an absolutely safe place near the temple to hide the key.
"I" wanted to hide the key in a place where no one knew and would not be shaken for thousands of years. As long as I didn't take it, I could hide it until the end of the world. But I couldn't throw the key into the lake or under the cliff. It had to be a place where "I" could take it if I wanted to.
It was not certain when I would come to take it, but this possibility must be retained. This possibility would connect me and it forever.
"I" painstakingly searched for the place where the key was hidden, but unexpectedly comprehended the old abbot's intention, so the two "people who hid things" came to one place across decades of time.
It turned out that decades ago, the master and his trusted apprentice went to the stream outside the temple, moved the small stone bridge and threw it into the mountain stream, and replaced it with the "蛱蝶碑".
Over the years, all kinds of people looking for the蛱蝶碑 have hurriedly passed by it, but no one has understood the truth, while the scriptures on the monument have silently carried and ferried ignorant sentient beings.
"I" walked to the bottom of the bridge in the dark, and with the help of the flashlight, I enjoyed the majestic and graceful words of the "蛱蝶碑", and for the last time, I held the key that connected to the old times of my hometown tightly and stuffed it into the gap of the bridge pier.
Of course, "I" didn't tell anyone, and the two secrets slept together.
Is Satoshi Nakamoto the only person in the world who knows Satoshi's private key?
When I thought of this question, a flash of lightning flashed through my mind.
Satoshi Nakamoto's 1.1 million bitcoins have now become the "stabilizing force" of the Bitcoin world. If they sleep forever, everything will continue.
Once one of the coins is moved, everyone will panic, because the resurrection of these "planets" means that Satoshi Nakamoto is not only alive, but can also sell all the bitcoins. There is no need to really sell them. This "possibility" is enough to collapse the consensus on the value of Bitcoin.
The fact is that Satoshi Nakamoto's wallet address has been as quiet as a dead volcano over the years.
The magma of those planets has solidified, and the window frames are silent and dim. Only from time to time, some Bitcoin believers throw some coins into them, as if they can get closer to the mysterious "creator" and pay tribute.
Have the living people, the dead people, and the unborn people in this world ever discovered or will they discover Satoshi Nakamoto's treasures?
If there is really an extremely smart person who cracks one of Satoshi Nakamoto's private keys, what is his best choice when facing the priceless Bitcoins on the planet?
Yes, of course, stop here for a moment, and then leave quietly, letting this secret continue to sleep in the surge of time.
Our world line will not be affected by this.
Only he knew that at that moment, he had looked into the eyes of a proud soul.







