Bitcoin’s Power Law Theory

Bitcoin resembles a natural phenomenon more than a normal asset. Credit for this beautiful art goes to: @BainterSAT

Bitcoin is more like a city and an organism than a financial asset.

My main finding is that Bitcoin is governed by a power law. Its laws suggest that it behaves more like a physical system than an asset. This intuition is based on observing a striking power law over multiple orders of magnitude in Bitcoin's price versus time.

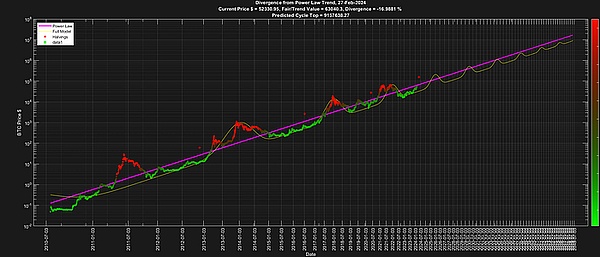
Bitcoin Power Law Theory

My power law model has now evolved into a complete theory of Bitcoin's behavior that can explain all major on-chain parameters and describe the growth of Bitcoin adoption in a scientifically coherent and falsifiable way: the Bitcoin Power Law Theory or PLT.

The figure below explains the Bitcoin power law theory in a nutshell and shows the main data supporting it. Price, hash rate, and addresses (we use addresses above a cutoff to eliminate dust addresses) are all power laws of each other and time. They all interact and influence each other in a continuous feedback loop.

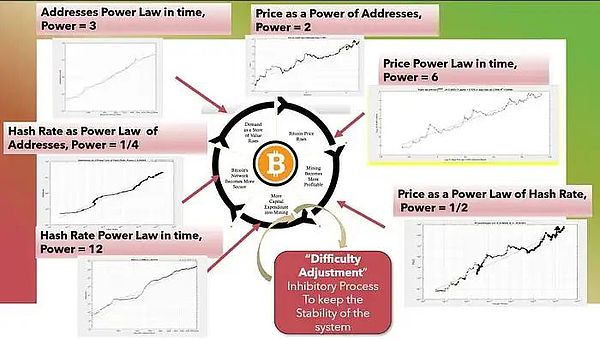
Power laws are mathematical expressions of the form y=A x^n and are ubiquitous not only in nature, but also in social phenomena and many parameters related to the development of cities or countries.

They are so common because it can be proven mathematically and physically that they appear whenever there is some process where the output becomes a new input in an iterative process.

This is exactly what happens with Bitcoin, for example, the hash rate now affects the hash rate later in an infinite loop. So it is surprising, but also completely in line with the nature of Bitcoin, that its behavior is governed by a power law. The interaction is supported by the following graph, which is well known in the community. I did not invent this graph, but I use it to illustrate how the theory works.

The theory is basically a mathematical expression based on the logic, physics, and mathematics of the feedback loop below.

1. Initially, Bitcoin needs to be accepted and adopted by the first users in Satoshi's circle.

2. The "value" (the "price" that can now be viewed online 24/7) increases with the square of the number of users (the empirical measurement is more like 1.95, but for simplicity we round all the powers below to integers). This confirms a theoretical result called Metcalfe's Law.

(Note: Metcalfe's law (English: Metcalfe's law) is a law about the value of the network and the development of network technology. It was proposed by George Kidd in 1993, but named after the computer network pioneer Robert Metcalfe in recognition of his contribution to Ethereum. Its content is: the value of a network is equal to the square of the number of nodes in the network, and the value of the network is proportional to the square of the number of networked users.)

The law points out that the more users a network has, the greater the value of the entire network and each computer in the network.

3. Rising prices bring more resources and capabilities, especially mining capabilities.

4. The increase in price reduces the time to mine a block, but the hashrate required to mine a block changes iteratively due to the "difficulty adjustment". Since mining is barely profitable, the compensation mechanism needs to be proportional to the price increase P=users² increases, and the reward itself logically and dimensionally, we have hashrate=Price² (which is exactly what is observed in experience as hashrate values ​​approach 2 or Price=hashrate^1/2).

5. The increase in hashrate brings more security to the system, which attracts more users. Now some readers may say that most people do not buy Bitcoin because of "security", however their purchase indirectly increases the "security" of Bitcoin. Because if it were not a secure system, no one would invest huge value in it. So yes, the security of the system directly or indirectly brings new users.

6. Users grow in powers of 3 over time. This is also a new result of the theory. Most models of Bitcoin adoption assume an S-curve type of growth. S-curves are typical of the adoption of many technologies like televisions, refrigerators, cars, cell phones, etc. Bitcoin does not follow an S-curve that initially grows exponentially. It follows a power-law of 3 in time. It turns out that many phenomena have an underlying S-curve adoption or spread mechanism (e.g. viruses) that become power-laws if they have inhibitory mechanisms. In the case of Bitcoin, the "difficulty adjustment" and the risk involved in any type of investment are inhibitory mechanisms, which is why we empirically observe that the growth of Bitcoin adoption follows a power-law of 3. There is a large literature showing examples of this type of disease spread containment phenomenon when risks like AIDS are involved (Bitcoin is not AIDS, but these studies show that if the spread of a disease involves some kind of decision, like having sex, it makes the disease spread like a power of 3 in time, rather than an S-curve or other type of logistic curve). 7. The cycle repeats indefinitely. Bubbles are an important and necessary component of this cycle, and they are discussed separately in the corollary below.

8. This power-law adoption growth (together with the previously explained power laws) explains why we observe the other power laws in time: Addresses=t3, Price=Address2=(t3)2=t6, Hashrate=Price2=(t6)2=t12.

Here is a chart showing all the interacting power laws and their proposed causal explanations.

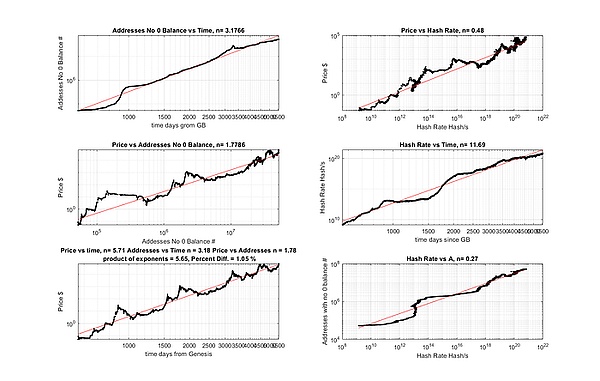
PLT Consequences and Predictions

This theory explains the long-term behavior of Bitcoin, and it has many consequences. The most surprising and relevant, and often misunderstood by most ordinary Bitcoin investors, is scale invariance.

Scale invariance is a property of objects or laws that remain the same when the scales of lengths, energies, or other variables are multiplied by a common factor. It is a feature used in physics, mathematics, and statistics.

Scale invariance is a typical feature of systems governed by power laws.

Essentially, it says that the system will continue to scale as it grows in the same way, which is why we can use scale invariance to make predictions, and given that the growth of the system has been expressed for over 9 orders of magnitude, it will continue to happen, almost certainly for another 1 or 2 orders of magnitude (it took about 10 years to reach 1 million BTC). While this sounds incredible, in the long run, all important information about the system, price, hash rate, and adoption is predictable.

Scale invariance also allows us to understand the role and importance of events, such as the recent inflows of investment into the Bitcoin system from large institutional ETFs. Scale invariance tells us that these events will not significantly affect Bitcoin's price trajectory, but they are key events necessary for the system to continue its scale-invariant growth. This also means that many people will find it difficult to understand that the power law trend (plus the bubble) is all you get. No more, no less. This is the most shocking prediction of the theory.

All theories are falsifiable, which is one of the ways to falsify theories, at least in their current form.

Future theories can be modified to add changes in slope or phase transitions, but in their current form, the theory says that the path of Bitcoin's price is already established and it will not change unless something catastrophic happens, especially for 1 or 2 orders of magnitude, which is only a small fraction of Bitcoin's overall historical growth. If it scales invariant for 15 years, it will probably continue to scale invariant for another 10 years (the next order of magnitude).

(Note: The author does not further explain why it will continue to be maintained for 10 years. This theory comes from the "Lindy Effect". The Lindy Effect believes that for things that do not age naturally (such as technology, culture, ideas, institutions, etc.), the longer it exists, the greater the possibility that it will continue to exist. In other words, if something has existed for a long time, then it is likely to continue to exist. If something has been maintained for 15 years, then according to the logical reasoning of the Lindy Effect, it is likely to continue for another 10 years (the next order of magnitude time period). This theory is mainly used to predict the lifespan of non-biological or non-organic things, such as Bitcoin, classic literature, philosophical thoughts, etc.)

By the way, in terms of scale, the next 10 years are not compatible with the previous 15 years because it is just another order of magnitude. For most people who are not familiar with these ideas, it takes some time to understand how logarithmic scaling works.

The principle of scale invariance for time prediction is simple. Keep everything proportional in log-log space (or scale space). It's as simple as making a triangle bigger and keeping all its sides proportional.

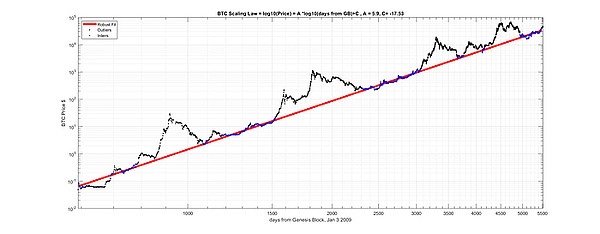
In the figure below, I applied this principle (pedagogy) to a prediction made by Harold Christopher Berger 5 years ago using power laws (blue dots). 5 years later, the prediction turned out to be correct (red dots). You can see that one could have used scale invariance to make a prediction (he did so indirectly by assuming the path continued). Scale invariance is used all the time in science to make predictions.

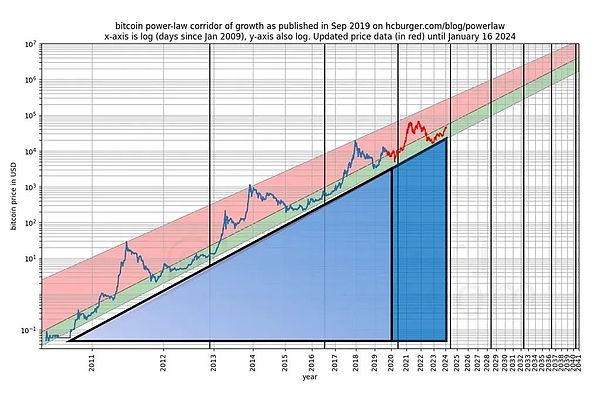
There's a lot more to the theory (e.g. why we see the bases that follow power laws aligned like this), but they will be elaborated on in subsequent posts.

I'm writing a scientific article (so I've peer reviewed these ideas and made sure they have scientific validity) and a popular book on the subject (in the style of my compatriot Galileo's "Dialogue Concerning the Two Chief World Systems").

Corollaries to the Theory

How the Bubble Works

It's not about scarcity, it's all about Moore's Law.

Satoshi knew about Moore's Law. It's a heuristic law that claims that computing power doubles every 2 years. The "difficulty adjustment" mechanism guarantees that you need to spend a lot of money and energy to get a few extra coins.

But Moore's Law gives you an unfair advantage. In 4 years you will have 4 times the hashing power, for essentially the same energy cost as a machine from 4 years ago (roughly). You need to update anyway due to wear and tear, and the cost of the machine is only part of the cost of running it. It turns out (and I explain how in my theory) that both logically and empirically we have price (or reward in general) = hashrate¹/2. So basically 4x the hashrate only gives you 2x the benefit. But then the halving cuts the benefit in half, leaving you with zero benefit. This is all designed to keep miners on the edge of profitability, never allowing for a free lunch. It's too perfect to be accidental, and I think Satoshi planned this for exactly that.

4 years instead of 2 or a constant reduction in rewards is there because it's also a good idea in terms of logistics, as it gives the chip industry time to update and advance, and also gives miners time to plan updates and let the equipment depreciate naturally. It's pure genius, very pragmatic, and hits the nail on the head about anything to do with Bitcoin. The bubble is the result of part of a "security attracts more adoption cycle". I didn't even invent this cycle, others did, and it's been used to explain the cycle of adoption ever since.

This makes sense because directly increasing security brings people in and gives you confidence in Bitcoin's ability to store value. Without that, there is no value. My best analogy is when people move to a growing city (Bitcoin is a shining city in the digital world, as Saylor calls it) when there is a burst of activity. You want to move in because of the bridges, the houses, the roads, etc. You don't necessarily think about those things directly, it's just that you are attracted to the activity. That's where all the new and good things happen. This creates a temporary FOMO, which is good FOMO because it's based on fundamentals, not some stupid speculation, maybe FOMO isn't the best word, so you can help me find a better word. But you get my point.

The price goes up very quickly, almost exponentially. This is the only time price does this, instead of growing like a power law. It overshoots due to all the excitement, but then it needs to go back, and in fact, you can see from the chart below that it is almost perfectly symmetrical, the price falls just as fast as it went up (sometimes faster). The bubble bursts and it goes back to equilibrium. It is a form of punctuated evolution and is necessary for Bitcoin to grow.

"Punctuated equilibrium is the idea that evolution moves in spurts, rather than following the slow but steady path proposed by Darwin. Long periods of stagnation with little activity in terms of extinction or the emergence of new species are interrupted by intermittent bursts of activity."

So bubbles are part of the Bitcoin story too. They are not the main story of the overall power law growth, but they are an important part of it and a necessary part of it.

I think this perfectly explains the entire cycle of bubble growth (about 2 years) and bubble period (about 2 years). Let me know what you think and if this makes sense.

This article by famous physicist D. Sornette has a very similar stance on the origin and properties of bubbles.

Scarcity plays no role in this theory at all. Scarcity has no mechanism or explanatory power.

Q&A

I don't understand what a power law is?

A simple concept, it is a relationship of the type y=A x^n. Despite the simplicity of this type of equation, it represents many phenomena in nature and man-made phenomena. But how can power laws appear in Bitcoin when Bitcoin was created through human interaction?

First, it’s not true that Bitcoin was created solely by human interaction. It’s code with a precise algorithm, after all, working with precise mathematical formulas. The “difficulty adjustment” is one of many feedback loops in the system, acting like a thermostat, so it can be studied as a physical system. The energy requirements of miners are also purely physical. But more social interaction-based physics, like the adoption of new Bitcoins, can also be modeled by equations similar to those found in physics and biology, like the spread of viruses.

Single individuals may have free will and act independently, but when you consider large numbers of agents, patterns emerge that can be studied using the tools we develop to understand natural phenomena. We call this universality, which means that we can find similar patterns in nature, independent of the specific nature of the phenomenon being studied.

Scientists have applied these methods to the growth of social networks, how cities grow, how businesses survive after completion, and so on. These social or economic phenomena often follow power laws. Even terrorist attacks follow power laws.

Why not use another currency besides the dollar?

Compared to most currencies in the world, the dollar is still stable. It's inflationary, but that's a small adjustment for Bitcoin. When we study physics, we first simplify and exclude possible complications, such as friction or air resistance. We can always add them later, but first, we want to understand the nature of the phenomenon without interference.

Do power laws apply to inflationary currencies?

I don't know, and why would I try? What information do we get from it? I could do it, but I want to explore 300 things about Bitcoin, and it seems like a waste of time, just like these currencies are wasteful. In general, though, the power law in BTC applies to stable inflation. If you start to have something that's too inflationary (like a rapidly increasing inflation rate), then the problem is not the power law, but the inflationary currency.

It's like I told you that gravity works and makes objects fall. Then you ask what about in a hurricane? Yes, a cow can fly during a hurricane, and so can you and your house. That doesn't violate the law of gravity.

Do you see the logical fallacy here?

When you create a scientific theory, in the case of Bitcoin, you always start with simple cases.

For example, use a stable number like the dollar, which "is" inflationary, but has a small constant exchange rate (at least compared to the peso). Then, as you understand simpler and more general cases, you can study more special cases. But at this point, you're not studying gravity, you're studying the lift created by a hurricane. That's a completely different phenomenon. People are so scientifically illiterate and lack critical thinking that it's really hard to convey basic ideas and concepts.

What will the price be in 2060? 10²⁹⁹⁹¹²³⁵ Are you happy now? BPLT should not be used for predictions beyond 2040 at best. Ray Kurzweil's technological singularity will follow and all predictions are shattered. History has a literal singularity, so no one knows what will happen. It can't go up forever. 1. We don't know because we don't know how much value will be transferred into Bitcoin in the future. We could start mining asteroids or invent nanotechnology and usher in a new era of abundance and wealth, so that Bitcoin could go up forever (see question above). 2. The model can be easily tweaked by adding a gradient component. Power laws are basically approximations of this model. By the way, these models do not lead to exponential behavior, but in fact, they are milder than power laws themselves. For now, there is no need to add this component that makes the model more complicated without actually benefiting our understanding.

You said price = hash rate ^ (1/2), but the equation does not have the correct dimensions.

For simplicity, we mean that the relationship is inherently proportional, and the correct equation is of course price = A hash rate ^ (1/2), where A is a constant with the correct units to make the equation work in dimensions.

In the early and middle stages of an outbreak, the growth of the virus usually follows an exponential pattern, not a power law. Obviously, the exponential spread of the virus will not continue indefinitely (through immunity, behavioral changes, vaccination, etc.), and may follow other growth patterns such as logarithmic growth patterns. It is important to emphasize here that this growth is not a power law. So that is not what we want to discuss today.

I will add the references as soon as possible. A large number of studies show that when there is a suppressive mechanism, the virus infection will become a power law. Prices are autocorrelated, therefore power laws are spurious. This is one of those arguments loved by statisticians and economics "experts". Of course, it is autocorrelated, and we claim it is deterministic. So you support our hypothesis? Anyway, there is a lot more that can be said about this ridiculous argument, which you can read in the linked article below, where we debunk the debunker.

Also, please note the following peer-reviewed article on Bitcoin, which makes a similar argument in a more polite and professional manner, that if you state at the outset that you are claiming causality because of a plausible mechanism, then you can ignore these more formal tests of causality, because if there is causality and the data are partially deterministic, then the data will be clearly correlated.

All of the power laws we observe are thought to be created by causal processes, like Metcalfe's law, "difficulty adjustment", power laws like social information propagation and interactions between users of the Bitcoin network. Therefore, we apply the same argument below, about the omission of these tests and their inappropriateness in the context of studying Bitcoin as a natural process (based on principles and mechanisms similar to biology, network theory, and physics).

What happens if the dollar falls into hyperinflation? Will the model break to the upside?

One ​​of the most common and annoying questions ever. What is the questioner trying to imply here? Will he be a millionaire soon?

1. What would be the benefit? Even more worthless dollars? Do you dream of this scenario? You would be a millionaire with a worthless currency. Happy?

2. Do you realize that this could lead to civil war, or even nuclear war? So what do you do with your Bitcoins? 3. If that happens, Bitcoin charts will be your last problem. All “models will be destroyed” Saylor wasn’t even referring to the Bitcoin model at all, but some general model about the economy. I had to go back and listen to that interview. Completely irrelevant. Then let’s stop and let people, including me, figure it out for themselves. You can. I love Saylor till death, but I’m sure he never drew Bitcoin charts himself, or if he did, he didn’t spend years studying them. When he talks about Bitcoin, I try to understand it. OK, let’s compromise, all models will be destroyed, and power law theory is a theory, not a model. Ok? Are we at the beginning of the S curve?

No, for several reasons (which we’ll discuss shortly).

If knowledge does become commonplace, will the value soar because people will price in the future?

No, that goes against one of the main predictions and fundamental principles of PLT. Any kind of momentary manipulation could make the price go up or down. But it can’t be sustained, and overall the trend will be respected.

It’s a hard concept to grasp. You can realize that the relationship between patents and city size is a power law, but you can’t change it, or change it too much is a fundamental property of the system. It doesn’t exist by accident. It’s what the system is all about. The power laws we observe in Bitcoin are what Bitcoin is. We can’t change them unless we fundamentally change Bitcoin. It’s the most powerful and important part of the theory, and it can be falsified over time, or more observations will support it.

References

1. Why Stock Markets Crash. Critical Events in Complex Financial Systems" Didier Sornette, 2002.

2. "Universal Cointegration and Its Applications" Tu et al., including supplemental information

3. Phillips, P . C. (1986). Understanding spurious regressions in econometrics. Journal of econometrics, 33(3), 311–340.

4. Scale: The Universal Laws of Life, Growth, and Death in Organisms, Cities, and Companies G. West

5. Bitcoin power law, over 10 year period, all the way to Genesis Block. : r/Bitcoin (reddit.com)

6.https://www.reddit.com/r/Bitcoin/comments/21pujs/bitcoin_compared_with_metcalfes_and_zipfs_law/